IJCRR

IJCRR - 9(24), December, 2017

Pages: 55-61

Date of Publication: 26-Dec-2017

Soret and Dufour Effects on MHD Casson Fluid Over a Vertical Plate in Presence of Chemical Reaction and Radiation

Author: N. Ananda Reddy, K. Janardhan

Category: General Sciences

Abstract:The combined effects of chemical reaction, radiation, Dufour and Soret effects on Casson MHD fluid flow over a vertical plate with heat source/sink has been studied with constant velocity, variable temperature and concentration. The governing equations of the flow have been solved by using the finite difference method. The effects of various parameters on velocity, temperature and concentration fields are studied with the help of graphs. It is found that velocity decreased with the increase of Casson parameter, Hartmann number, thermal conductivity, Schmidt number, Chemical parameter and decrease of Soret effect, Thermal
Grashof number and Mass Grashof number. Concentration found to be decreasing with the decrease of Soret number and increasing chemical parameter. Temperature decreased when thermal radiation is increased and heat source is decreased.

Keywords: Casson fluid, Dufour effect, Soret effect, MHD, Chemical reaction

Full Text:

Introduction

Casson fluid exhibits yield stress. It is well known that Casson fluid is a shear thinning liquid which is assumed to have an infinite viscosity at zero rate of shear, a yield stress below which no flow occurs, and a zero viscosity at an infinite rate of shear, i.e., if a shear stress less than the yield stress is applied to the fluid, it behaves like a solid, whereas if a shear stress greater than yield stress is applied, it starts to move. Casson fluids like Honey, human blood, jelly, sauces, etc., are having significant importance. Human blood can also be treated as Casson fluid.

In recent days, Casson fluids have occupied a prominence role in various fields like biological, chemical, medical and engineering applications. in industrial environment, Various researchers have carried out their research work in this direction  enlightening  the effects of various parameters on the flow of the Casson fluid.Mustafa and Khanstudied the MHD flow and heat transfer of Casson nanofluid over a non-linearly stretching sheetwith non-linear temperature distribution.The heat and mass transfer characteristics of a magneto hydrodynamic Casson fluid flow on a parallel plate channel having stretching walls, when subjected to a uniform transverse magnetic field are analyzed by Sarojamma et al. Swati et al numerically examined the boundary layer flow due to an exponentially stretching surface in the presence of an applied magnetic field. Casson fluid model is used to characterize the non-Newtonian fluid behavior. The flow issubjected to suction/blowing at the surface. Analysis is carried out in presence of thermal radiation and prescribed surfaceheat flux. Prakash et al studied the MHD two-dimensional boundary layer flow of a Casson fluid in the presence of chemical reaction and thermal radiationaccompanied by heat and mass transfer towards an exponentially stretching sheet.

Animasaun et al. studied the thermal conductivity and motion of temperature dependent plastic dynamic viscosityof steady incompressible laminar free convective MHD Casson fluid flow over an exponentially stretching surface with suction and exponentially decaying internal heat generation. The effects of thermal radiation, suction/blowing, viscous dissipation, heat source/sink and chemical reaction onboundary layer flow of a non-Newtonian Casson fluid in presence of heat and mass transfer towards a porous exponentially stretching sheet with velocity slip and thermal slipconditions were analyzed by Saidulu and Lakshmi. The steady flow and heat transfer of Casson fluid from a permeable horizontal cylinder in the presence of slip condition in a non-Darcy porous medium is analyzed by maintaining the cylinder surface at a constant temperatureis studied by Prasad et al. Shehzad et al derived a series solution for the effect of mass transfer in the MHD flow of a Casson fluid over a porous stretching sheet in the presence of a chemical reaction. Casson fluid flow over a vertical porous surface with chemical reaction in the presence of hydromagnetic field has been studied by Arthur et al. The heat and mass transfer effect in a boundary layer flow of an electrically conducting viscous fluid subject to transverse magnetic field past a moving vertical plate through porous medium in the presence of heat source and chemical reaction is analysed by Tripathy et al.

The aim of the present paper is to analyze the combined effects of chemical, radiation, Dufour and Soret parameters on Casson MHD fluid flow over a vertical plate with heat source/ sink has been studied with constant velocity, variable temperature and concentration. The governing equations of the flow have been solved by using the finite difference method. The effects of varies parameter on velocity, temperature and concentration fields are studied with the help of graphs.

Formulation of the problem:

Consider a two-dimensional steady Casson fluid of incompressible, viscous, electrically conducting fluid over a vertical plate moving with constant velocity with radiation and chemical reaction in the presence of Soret and Dufour is considered. Consider the flow of an incompressible viscous fluid passing a flat sheet coinciding with plane y = 0.We select the Cartesian coordinate system such that the x − axis be taken parallel to the surface and y is perpendicular to the surface. The fluid occupies a half space y>0. The flow is subjected to a constant applied magnetic field B₀ in the y-direction. The magnetic Reynolds number is considered to be very small so that the induced magnetic field is negligible in comparison to the applied magnetic field. The surface temperature of the plate oscillates with small amplitude about a nonuniform mean temperature. The fluid is assumed to have constant properties except for the influence of the density variations with temperature and concentration which are considered only in the body force term. The temperature of the plate oscillates with little amplitude about a non-uniform temperature.

The rheological equation of state for an isotropic flow of a Casson fluid [000]can be expressed as:

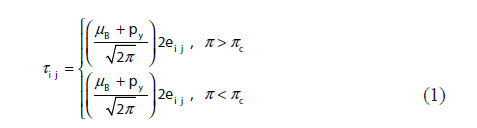
In Equation (1), =e_{i j} e_{i j}, where e_{i j}is the (i , j)^th component of deformation rate. This means that is the product of the component of deformation rate with itself. Also,  is a critical value of this product based on the non-Newtonian model, 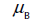 is the plastic dynamic viscosity of the non-Newtonian fluid and P_y is the yield stress of the fluid. The equations governed the unstudied boundary layer flow of the Casson fluid is

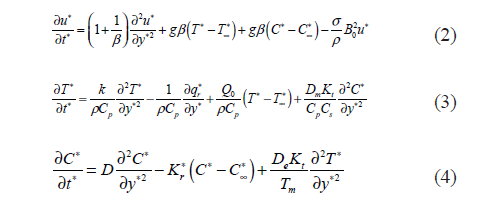
Equations (2),(3) and (4) refers Momentum Equation, Energy Equation and Species Equation respectively where u is the velocity of the fluid, b is Casson parameter, Q₀ is the heat source/sink parameter, D is the molecular diffusivity, k is thermal conductivity, C is mass concentration, t is time, υ is the kinematics viscosity, g is the gravitational constant, b and b* are the thermal expansions of fluid and concentration, ρ is density, c_p is the specific heat capacity at constant pressure, Dm Coefficient of mass diffusivity, kt Thermal diffusion ratio, Tm Mean fluid temperature, T∞ Free stream temperature of the surrounding fluid, C∞ Free stream concentration, T Fluid temperature, C Fluid concentration y is distance, qr is the radiative flux, b is the magnetic field, kr is the chemical reaction rate constant.

R.H.S. of equation (2), second term is thermal heat effect,third term is thermal concentration effect, fourth term is magnetic effect, and second term is thermal buoyancy effect. R.H.S. of equation (3) second term is thermal radiation flux and third term is thermal radiation and fourth term is Dufour effect. R.H.S. of equation (4), second term is chemical reaction and third term Soret effect. Under the above assumptions the physical variables are functions of y and t. The boundary conditions for the velocity, temperature and concentration fields are:

Introducing the dimensionless quantities with thermal radiation flux gradient expressed and we assume that the temperature differences within the flow are sufficiently small so that  can be expressed as a linear function of  after using Taylor’s series to expand  about the free stream temperature 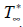 and neglecting higher-order terms. This results in the following approximation.

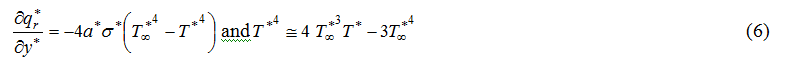
The following dimensionless quantities are introduced

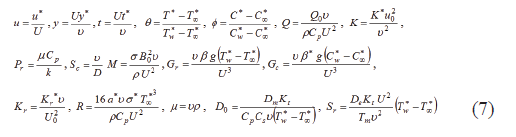
The thermal radiation flux gradient may be expressed as follows

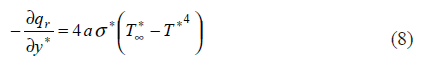
Considering the temperature difference by assumption within the flow are sufficiently small such that   may be expressed as a linear function of the temperature. This is attained by expanding in  Taylor’s series about  and ignoring higher orders terms.

Substituting the dimensionless variables (7) into (2) to (4) and using equations (8) and (9), reduce to the following dimensionless form.

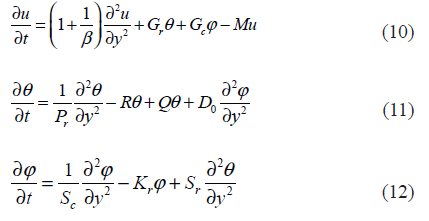
The corresponding boundary conditions are

Where G_r is thermal Grashof number, P_r is the prandtl number, k_r is the chemical reaction parameter, R is the thermal radiation conduction number, M is Hartmann number, G_c is the mass Grashof number, Q is the heat source/sink parameter and S_r is the Soret number.

Method of Solution

Equations (10)-(12) are linear partial differential equations and are to be solved with the initial and boundary conditions (13). In fact the exact solution is not possible for this set of equations and hence we solve these equations by finite-difference method. The equivalent finite difference schemes of equations for (10)-(12) are as follows:

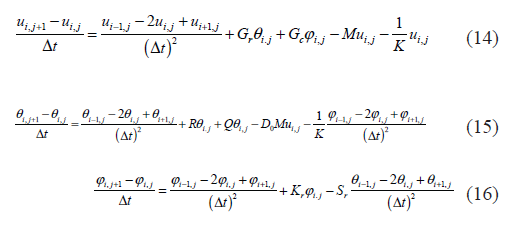
Here, the suffix ‘i’ refer to y and ‘j’ to time. The mesh system is divided by taking ?y = 0.1. From the initial condition in (13), we have the following equivalent:

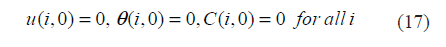
The boundary conditions from (13) are expressed in finite-difference form as follows

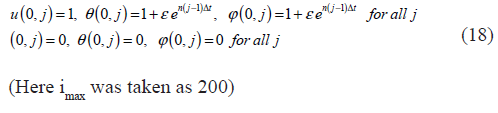
The velocity at the end of time step viz, u(i, j+1)(i=1,200) is computed from (14) in terms of velocity, temperature and concentration at points on the earlier time-step. After that θ (i, j +1) is computed from (15) and then C (i, j +1) is computed from (16). The procedure is repeated until t = 0.5 (i.e. j = 500). During computation ?t was chosen as 0.001.

Skin-friction:

The skin-friction in non-dimensional form is given by the relation

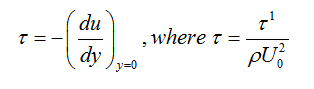
Rate of heat transfer:

The dimensionless rate of heat transfer in terms of Nusselt number is given by

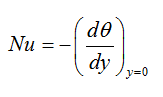
Rate of mass transfer:

The dimensionless rate of mass transfer in terms of Sherwood number is given by

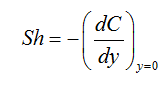
Results and Discussion:

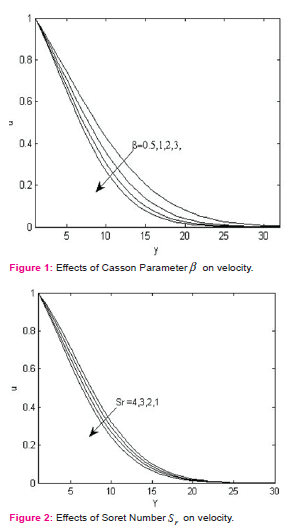
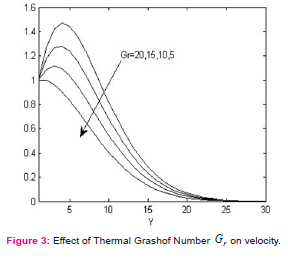
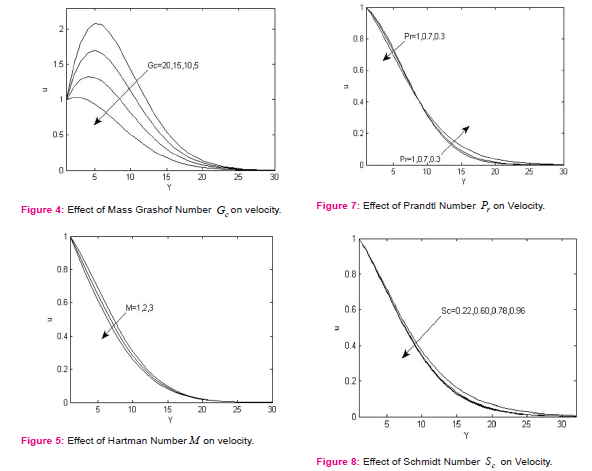
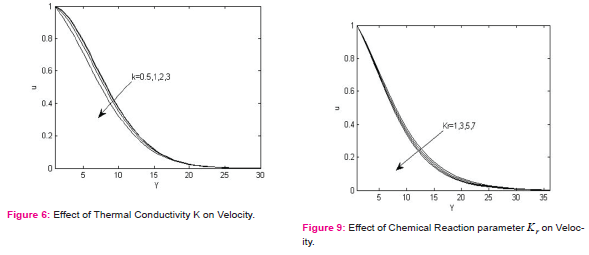
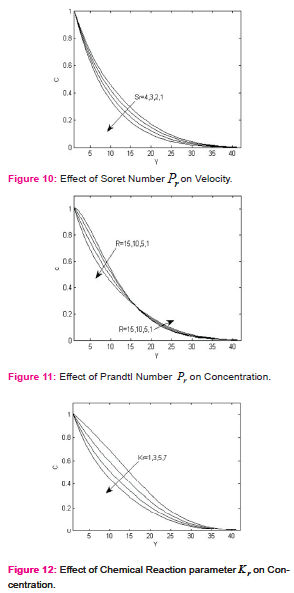
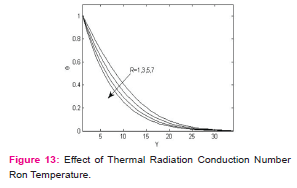
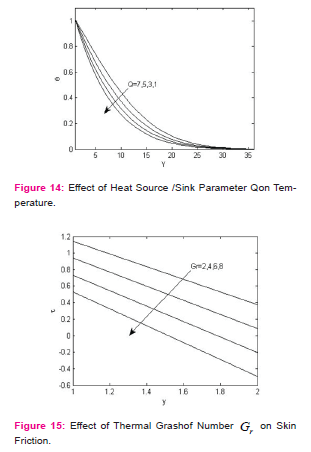
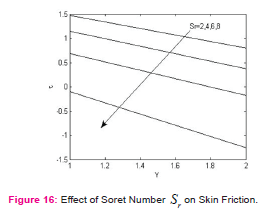
The effects of various parameters such as Casson Parameter “ ”, Thermal Grashof Number “G_r”, Mass Grashof Number “G_r”, Thermal Conductivity “K”, chemical reaction parameter k_r, Prandtl Number “P_r” Schmidt Number “S_c” Thermal radiation conduction number “R”, Hartmann number M, Heat source/sink parameter Q and Soret number S_r on the velocity, temperature and concentration fields are studied numerically and represented the results through graphs. The influence of Casson parameter, Hartmann number, thermal conductivity, Schmidt number and chemical reaction parameter on velocity is shown in the figures 1,5,6,8,9. From these figures, it is recognized that the velocity decreases with the increasing of these parameters respectively. The influence of Soret number, thermal Grashof number, the mass Grashof number on velocity are shown in the figures 2,3,4. It is clear that the velocity decreases with the decrease of these parameters respectively. The effect of Prandtl number on the velocity is shown in the figure 7. It is observed that the velocity decreases near the plate and increases far away the plate with the falling of the Prandtl number. The effect of Soret number on the concentration field is illustrated in figure 10. As the Soret number decreases the concentration is found to be decreasing. The effect of chemical reaction parameter on the concentration field is illustrated in figure 12. It is clear that the concentration is decreasing for increasing chemical reaction parameter. The effect of thermal radiation conduction number on concentration field is shown in figure 11. It is noted that the concentration decreases near the plate and increases far away the plate with falling thermal radiation conduction number. Figure 13 shows the variations of thermal radiation conduction number on temperature. It is found that the temperature is decreased when R is increased. Figure 14 shows the variation of the heat source sink parameter on temperature. It is clear that the temperature decreases when Q decreases. In Figure 15, the skin friction decreases when thermal Grashof number increases and figure 16 shows that the skin friction decreases when Soret number increases.

CONCLUSION

In this paper, from the study of the combined effects of chemical reaction, radiation, Dufour and Soret effects on Casson MHD fluid flow over a vertical plate with heat source / sink, it is concluded that

• Velocity decreased with the increase of Casson parameter, Hartmann number, thermal conductivity, Schmidt number, Chemical parameter and decrease of Soret effect, Thermal Grashof number and Mass Grashof number.
• Concentration is found to be decreasing with the decrease of Soret number and increasing chemical parameter.
• Temperature is decreased when thermal radiation is increased and heat source is decreased
• Skin friction decreased when thermal Grashof number and Soret number are increased

ACKNOWLEDGEMENT

Authors acknowledge the immense help received from the scholars whose articles

are cited and included in references of this manuscript. The authors are

also grateful to authors / editors / publishers of all those articles, journals

and books from where the literature for this article has been reviewed and

discussed.

References:

M. Mustafa, and Junaid Ahmad Khan , Model for flow of Casson nanofluid past a non-linearly stretching sheet considering magnetic field effects, AIP Advances5, 077148 (2015); doi: 10.1063/1.4927449

G. Sarojamma,  B.Vasundhara, K. Vendabai,  MHD Casson Fluid Flow, Heat and Mass Transfer in a Vertical Channel with Stretching Walls , International Journal of Scientific and Innovative Mathematical Research (IJSIMR) Volume 2, Issue 10, October 2014, PP 800-810 

Swati Mukhopadhyaya, Iswar Chandra Moindala, and Tasawar Hayat, MHD boundary layer flow of Casson fluid passing through an exponentially stretching permeable surface with thermal radiation, Chin. Phys. B Vol. 23, No. 10 (2014) 104701, DOI: 10.1088/1674-1056/23/10/104701

S. A. Shehzad, T. Hayat, M. Qasim and S. Asghar , Effects of mass transfer on mhd flow of casson fluid with chemical reaction and suction, Brazilian Journal of Chemical Engineering, Vol. 30, No. 01, pp. 187 - 195, March, 2013.

J. Prakash, P. Durga Prasad, G. Vinod Kumar, R. V. M. S. S. Kiran Kumar and S. V. K. Varma, Heat and Mass Transfer Hydromagnetic Radiative Casson Fluid Flow over an Exponentially Stretching Sheet with Heat Source/Sink, International Journal of Engineering Science Invention, Volume 5 Issue 7, July 2016, pp.12-23

I.L. Animasaun, E.A. Adebile,  A.I. Fagbade, Casson fluid flow with variable thermo-physical property along exponentially stretching sheet with suction and exponentially decaying internal heat generation using the homotopy analysis method, Journal of the Nigerian Mathematical Society 35 (2016) 1–17, http://dx.doi.org/10.1016/j.jnnms.2015.02.001

N Saidulu and A Venkata Lakshmi, Slip Effects on MHD Flow of Casson Fluid over an Exponentially Stretching Sheet in Presence of Thermal Radiation, Heat Source/Sink and Chemical Reaction, European Journal of Advances in Engineering and Technology, 2016, 3(1): 47-55

Ramachandra Prasad V, Subba Rao A and Anwar Bég O, Flow and Heat Transfer of Casson Fluid from a horizontal Circular Cylinder with Partial Slip in non-Darcy porous Medium, J Appl Computat Math 2013, 2(2), http://dx.doi.org/10.4172/2168-9679.1000127

Emmanuel Maurice Arthur, Ibrahim Yakubu Seini, Letis Bortey Bortteir, , Analysis of Casson Fluid Flow over a Vertical Porous Surface with Chemical Reaction in the Presence of Magnetic Field. Journal of Applied Mathematics and Physics, (2015) 3, 713-723.

http://dx.doi.org/10.4236/jamp.2015.36085

R.S. Tripathy , G.C. Dash , S.R. Mishra , S. Baag, Chemical reaction effect on MHD free convective surface over a moving vertical plate through porous medium, Alexandria Engineering Journal (2015) 54, 673–679,  http://dx.doi.org/10.1016/j.aej.2015.04.012