IJCRR

IJCRR - 4(13), July, 2012

Pages: 25-38

Date of Publication: 18-Jul-2012

RECENT DEVELOPMENTS IN ENHANCEMENT OF HEAT TRANSFER THROUGH ARTIFICIAL ROUGHNESS IN SOLAR AIR HEATER DUCTS

Author: Aditya Takhalate, A. R. Jaurker

Category: Technology

Abstract:The heat transfer between duct plate and air is low, which leads to higher temperature loss to atmosphere
in solar air heaters which result in lower performance of system. It is found that the application of artificial roughness breaks the viscous sub layer in ducts, decrease the thermal resistance and increase the turbulence in flow which enhances the heat transfer rate. The roughness geometry with their parameters
and correlations developed by various investigators are tabulated in this paper for suitable selection.

Keywords: solar air heater, artificial roughness, heat transfer, friction factor

Full Text:

INTRODUCTION
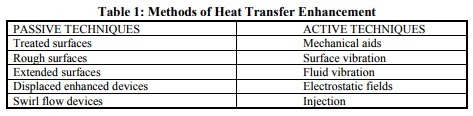
A solar air heater generally consists of an absorber plate forming a high aspect ratio through which air to be heated. It is more popular because it utilizes both the beam and diffuse radiation and simple in design, requires little maintenance. The absorber plate is a metal sheet about 1mm in thickness, usually made of GI or steel painted black to obtain higher absorbtivity. Transparent glass sheet having higher transmissivity of thickness 4 to 5 mm is the most commonly used cover plate of the top of the solar air heater. To avoid the heat losses, the sides and bottom are insulated with insulating material like mineral wool or glass wool of thickness 5 to 8cm. Since the heat transfer coefficient of air heater is low which can be improved by active/ passive techniques as listed in table 1. Artificial Roughness: Artificial roughness is the passive technique of heat transfer enhancement. Further a general classification of ribs according to modifications is listed in table 2.These are defined by non-dimension parameters as follows:- 1) Relative roughness pitch (p/e): It is defined as the ratio of distance between two consecutive ribs to the height of the rib. 2) Relative roughness height (e/D): It is defined as the ratio of rib height to the hydraulic diameter of the duct. 3) Angle of attack (α): It is defined as the inclination of rib with the direction of flow of air. Three main types of artificial roughness are generally found in literature as follows:

1. Three Dimensional Roughness (Uniform Roughness)
2. Ridge Type Two Dimensional Roughness (Repeated Ribs)
3. Groove Type Two Dimensional Roughness

1. According to Orientation of ribs

i. Transverse ribs

ii. Inclined ribs

iii. Helical ribs

2. According to Cross sectional views

i. Circular

ii. Rectangular

iii. Square

iv. Triangular

v. Trapezoidal

3. Discrete ribs or Staggered ribs

i. Transverse Discrete ribs

ii. Inclined Discrete ribs

iii. V – shaped Discrete

iv. W shaped Discrete

4. Grooves

5. Combined/ compound ribs

i. Transverse and Inclined ribs

ii. Rib grooves

iii. Special shaped rib and grooves

6. Dimple roughness

7. Special Shaped Ribs

i. V- shaped ribs

ii. W-shapes ribs

iii. Wedge Shaped ribs

iv. Chamfered

v. Arc shaped wire and

vi. Multi

V ribs

vii. Expanded metal mesh

RouRoughness in the form of sand grainsghness in the form of sand grains Nikuradse [1] investigated the effect of roughness on the friction factor and velocity distribution in pipes roughened by sand blasting. Nunner [2] and Dippery and Sebersky [3] developed a friction similarity law and a heat momentum transfer analogy for flow in sand grain roughened tubes.

Roughness in the form of transverse ribs
Ravigururajan and Bergles [4] developed general statistical correlations for heat transfer and pressure drop for four types of roughness, namely semicircular, circular, rectangular and triangular for single-phase turbulent flow in internally ribbed tubes. Han [5] carried out an experimental study in square duct with two opposite rib roughened walls. Son et al. [37] carried out the Particle Image Velocity (PIV)experiments for heat transfer characterization in two-pass square channels with smooth and 90° ribbed walls for high Reynolds number turbulent flow (Re= 30,000).The rib-induced flow turbulence increases the heat transfer mainly because of the enhanced local flow impingement near the rib.

Square transverse, Helically Ribbed, Trapezoidal, Three-Dimensional Ribs.
Firth and Meyer [35] investigated the heat transfer and friction factor performance based on equal pumping power, of four different types of artificially roughened surfaces, namely (i) Square transverse ribbed surface, (ii) Helically ribbed surface. (iii)Trapezoidal ribbed and (iv) Three-dimensional ribs. They found that the helically ribbed surface has a thermal performance which compares closely with the square transverse ribbed surface.

Rectangular, Semicircular and Square cross section ribs
Tanda [11] carried out experimental investigation by using liquid crystal thermography to study of heat transfer from a rectangular channel having width to height ratio of 5 and one surface heated at uniform heat flux and roughened by repeated ribs. The ribs having rectangular or square sections were fixed transverse to the main direction of flow or Vshaped with an angle of 45 or 60 deg relative to flow direction. The results show that for the range of Reynolds number studied, the presence of semicircular, rectangular and square ridges at two walls yield about 4-8 fold, 5-10 fold and 7- 15 fold increase in the average friction factor respectively relative to smooth duct. There is about 1.6 to 2.1 fold, 1.7 to 2.2 fold and 1.9 to 2.7 fold increase in the average heat transfer coefficient compared with smooth wall for the semi circular, triangular and square ridge walls respectively.

Square, triangular and trapezoidal
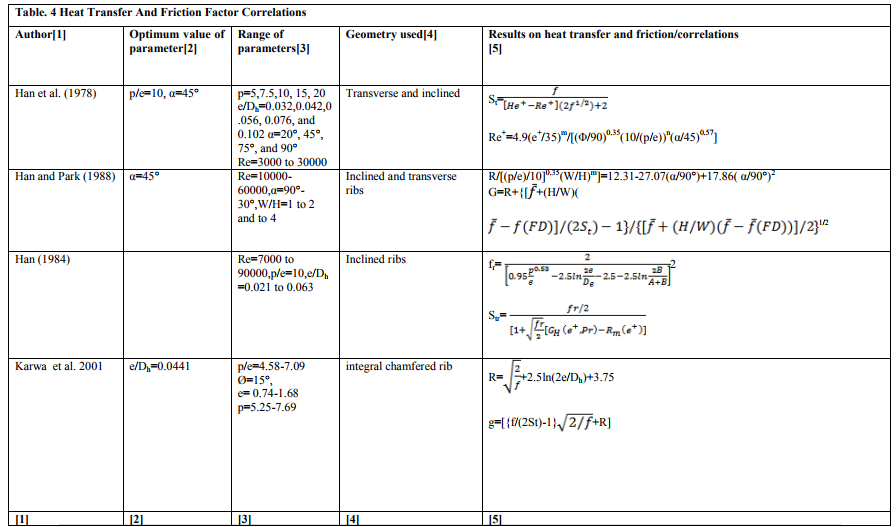
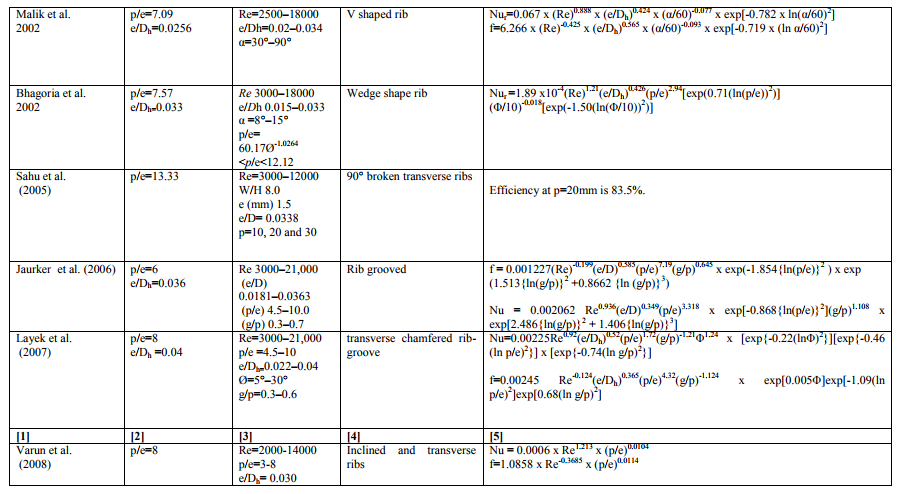
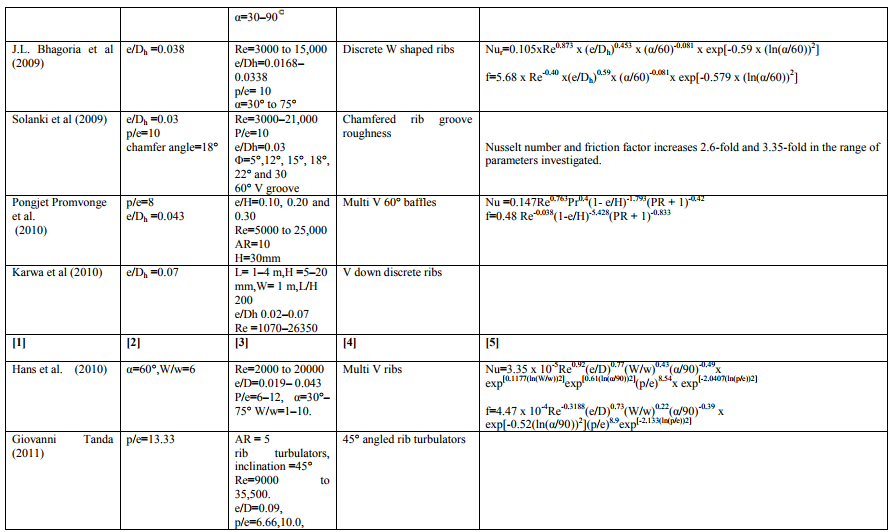
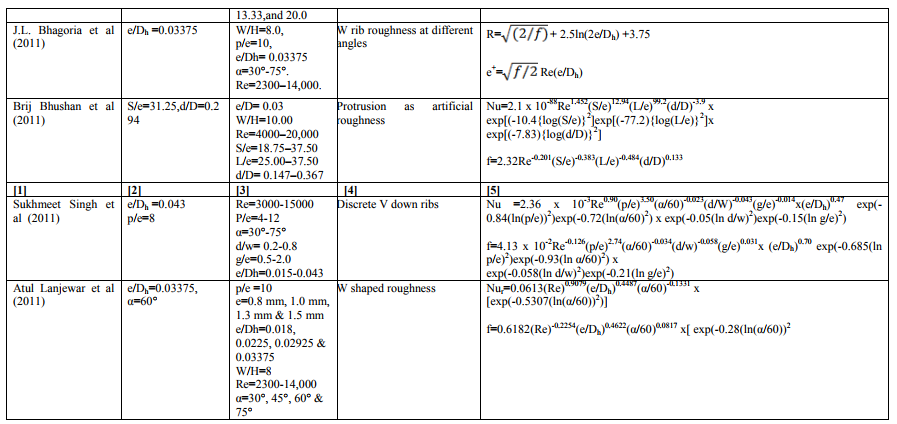
Kamali et al. [18] investigates the effect of square, triangular and trapezoidal ribs with computer code using square duct and the simulation was performed on all four ribs with parameters as relative roughness pitch varies from 8 to 12 and relative roughness height of 0.1 and it was found that the relative roughness pitch of 12 provides the highest heat transfer. Chamfered Ribs Layek et al.[14] used chamfered rib groove roughness on one wall of the solar air heater by varying parameters selected and it was found that highest Nusselt number occurs for chamfer angle of 18° but the friction factor increase with chamfer angle. The nusselt number increases 3.24 times and friction increases 3.78 times respectively. The maximum heat transfer enhancement was observed at relative roughness pitch of 6 and relative groove position of 0.4. Again Layek et al.[23] in the next paper investigates the effect of chamfer angle on heat transfer with chamfer angle as 5°, 12°, 15°, 18°, 22° and 30°.It was resulted that the maximum heat transfer occurs at chamfer. 90° Broken Transverse Ribs Sahu et al.[12]investigated heat transfer on transverse ribs with pitch 10-30mm rib height 1.5mm and it was found that the solar air heater provides maximum efficiency at a pitch of 20mm and heat transfer enhances to 1.4 times as compared to smooth duct. Inclined Ribs Han et al. [6] developed a general correlation based on the law of the wall similarity and the application of the heat-momentum analogy for friction and heat transfer to account for rib shape, spacing and angle of attack. In the experimental program, sixteen different geometries were tested for relative roughness pitch of 5,7.5,10,15,and 20, relative roughness height of 0.032,0.042, 0.056, 0.076, and 0.102 and angle of attack of 20°, 45°, 75°, and 90° and Reynolds number from 3000 to 30000. The main conclusions of the investigation are as : For small value of relative roughness pitch (p/e = 5), the flow which separates on each rib does not reattach before it reaches the succeeding rib while a relative roughness pitch value of about 10, the flow does reattach close to the next rib. The maximum value of both the heat transfer coefficient and the friction factor occur at relative roughness pitch (p/e) of 10, and ribs at a 45° angle of attack have superior heat transfer performance at given friction power when compared to ribs at a 90° angle of attack or when compared to sand-grain roughness. Angled Rib Turbulators Tanda et al.[27] employed inclined ribs to investigate heat transfer in rectangular channel by using it in one and two walls of the channel. For one ribbed wall the optimum performance was found at relative roughness pitch of 13.33 while for two ribbed wall the optimal performance was observed at relative roughness pitch of 6.66-10 for an angle of attack of 45°. Inclined Discrete ribs Aharwal et al. [21] used inclined discrete ribs on absorber plate and the effect of width and gap position has been investigated under the parameters as Reynolds number from 3000 to 18,000 with the relative roughness pitch (P/e) range of 4–10, relative roughness height (e/D) range of 0.018–0.037, and angle of attack (α) ranges from 30–90°. Result shows that Nusselt number increases 2.83 times and friction factor 3.60 times under the range of parameters investigated. The maximum heat transfer occurs at the relative gap position of 0.25 with the relative gap width of 1.0 for the relative roughness pitch of 8.0, angle of attack of 60° and relative roughness height of 0.037. The maximum value of friction factor occurs for discrete transverse ribs with relative roughness pitch of 8.0. Combined transverse and inclined ribs Varun et al.[15] investigates the effect of combined transverse and inclined ribs on absorber plate with Reynolds number (Re) ranges from 2000 to 14 000, relative roughness pitch (p/e)ranges from 3–8 and relative roughness height e/Dh= 0.030.Results shows relative roughness pitch of 8 have the maximum thermal efficiency. Again Varun et al [19].shows the effect of inclined and transverse ribs and optimized the efficiency of solar air heater by taguchi method and the maximum value of effective efficiency has been found for relative roughness pitch (p/e) of 8 with parameters as Reynolds number (Re) 2000–14,000, relative roughness pitch (p/e) 3–8 and a fixed value of relative roughness height (e/D) of 0.030. V Shaped Lau et al. [7] observed that the average Stanton number is 60, 45 or 30 deg discrete rib case is about 25 to 35 percent higher than in the 90 deg discrete rib case. In all the above configurations, the crossed ribs were found to perform poorly compared with other configurations. Momin et al. [9] investigated that for relative roughness height of 0.034 and for angle of attack of 60°, the V-shaped ribs enhance the values of Nusselt number by 1.14 and 2.30 times over inclined ribs and smooth plate at Reynolds number of 17034. Wedge shaped ribs Bhagoria et al.[10] used wedge shaped ribs with parameters as Re= 3000–18000,relative roughness height (e/Dh) varies from 0.015– 0.033,Relative roughness pitch 60.17Φ - 1.0264 turbulence is larger for the ribbed-grooved wall than for the ribbed wall. The additional vortices created by the grooves are responsible for the higher turbulence in the ribbed-grooved wall. Jaurker et al. [13] investigated the characteristics of rib-grooved artificial roughness. The parameters having Reynolds number range from 3000 to 21,000, relative roughness height 0.0181–0.0363, relative roughness pitch 4.5– 10.0 and groove position to pitch ratio 0.3–0.7. The effect of important parameters on the heat transfer coefficient and friction factor has been discussed. The results shows nusselt number increases 2.7 times and the friction factor increases up to 3.6 times.

U Shaped ribs
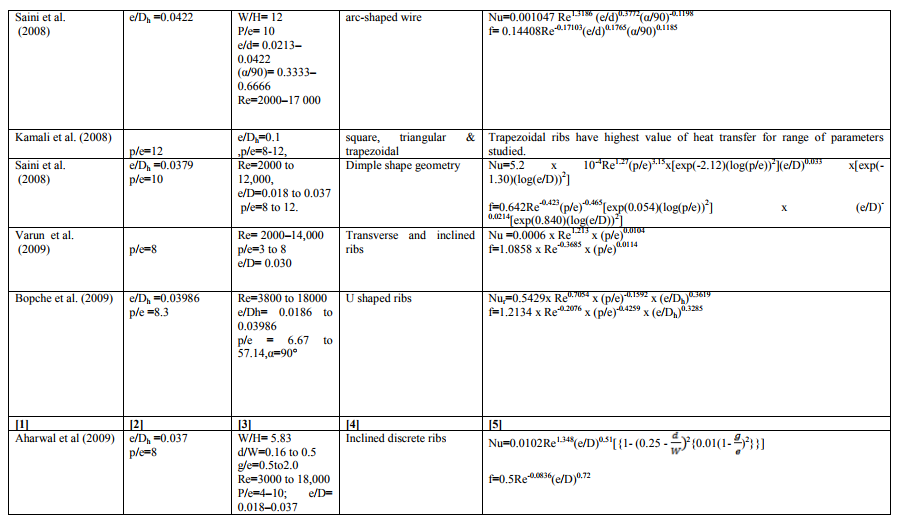
Bopche et al. [20] used U shaped turbulators on the absorber plate of solar air heater. It was found that the heat transfer increases 2.82 times while friction increases 3.72 times as compared to that of smooth duct. Multi V ribs Hans et al. [26] investigated multiple V ribs and the presence of this rib provides increase in nusselt number and friction factor of 6 and 5 times respectively. The maximum heat transfer occurs at relative roughness width of 6 while maximum friction occurs at relative roughness width of 10 and it was also resulted that the maximum enhancement in nusselt number and friction was found at angle of attack of 60°.Promvonge et al.[24] used multi 60 V baffles on absorber plate to find out the enhancement of heat transfer and it was found that thermal performance with PR=1 and e/H=0.10 results maximum thermal enhancement factor of about 1.87 at lower Reynolds number.

V Discrete
Gao and Sunden [36] carried out an experimental investigation on heat transfer and pressure drop in the cases of two wide rib roughened walls of rectangular duct with three rib configurations parallel ribs and V-shaped ribs pointing upstream or downstream of the main flow direction. The system parameters include e/Dh of 0.06, α of 60 degree and p/e of 10 for the Re ranges from 1000 to 6000.The Vribs pointing downstream produced the highest heat transfer enhancement and friction factors and provided the best thermal performance over the Reynolds number range tested. Parallel ribs provided better performance than that of V-ribs pointing upstream at high Reynolds numbers. Karwa et al. [25] investigated the effect of V down discrete ribs by using a mathematical model. And it was found that at sufficiently lower mass flow rates i.e. less than 0.04kgs-1m 2 the thermal and effective efficiency differ marginally but at higher mass flow rates i.e. greater than 0.045 kgs-1m 2 the thermal efficiency is higher than effective efficiency. Singh et al.[30] investigated experimentally the effect of V down discrete ribs on one wall of the duct. The maximum heat transfer is 3.04 times and friction factor is 3.11 times higher to that of smooth duct.

W shaped ribs
Lanjewar et al.[31] used W shaped ribs to increase the performance of air heater and it was resulted that heat transfer increases 2.36 and friction factor increases 2.1 times at an angle of attack of 60°.Kumar et al.[22] employs discrete W ribs on absorber plate having aspect ratio of 8:1.The maximum heat transfer occurs at 30°, 45°, 60° and 75° e/Dh of 0.0168 but at e/Dh of 0.0338 the maximum heat transfer was 1.88, 1.99, 2.16 and 2.08 times as compared to smooth duct. It was also resulted hat there was decrease in friction with increase in reynolds number. The effect of orientation of W shaped ribs on heat transfer was observed by lanjewar et al.[28] and it was found that better thermo hydraulic performance is obtained at α=60°.And it was concluded that W down ribs gives better performance than W up and V ribs.

Dimple shape geometry
Saini et al. [17] used dimple shape geometry as artificial roughness with parameters as Reynolds number 2000 to 12,000, relative roughness height of 0.018 to 0.037 and relative roughness pitch of 8 to 12.The maximum heat transfer was obtained at the relative roughness height of 0.0379 and relative pitch of 10.And the minimum friction was found at relative roughness height of 0.0289 and relative roughness pitch of 10.

ARC shaped wire
Saini et al. [16] investigates the effect of arc shaped wire as artificial roughness on duct and increase in heat transfer is 3.80 time while friction factor increases 1.75 times. The maximum heat transfer occurs at relative arc angle of 0.3333 and relative roughness height of 0.0422.

CONCLUSION
It is found that most of the investigators developed their correlations experimentally for the solar air heaters ducts having different geometries as artificial roughness in order to enhance the heat transfer. But some researchers have attempted to numerically model the roughness effects. And it was observed that it is the most promising technique to improve the performance of solar air heaters. Nomenclature Dh hydraulic diameter of duct,m e rib height, mm g groove position, mm h heat transfer coefficient, W/m2K H height of air duct, mm P pitch, m W width of duct, m Dimensionless Parameters d/D Relative print diameter d/W relative gap position e + roughness reynolds number e/D, e/Dh relative roughness height e/H rib to channel height ratio f friction factor G (e+ ) Heat transfer function GH Heat Transfer Roughness Function g/p relative groove position L/e relative long way length of mesh p/e relative roughness pitch Re Reynolds number R (e+ ) momentum transfer function R Roughness function St Stanton number S/e relative short way length of mesh W/H Aspect Ratio Greek Symbols α angle of attack Φ wedge angle α/90 arc angle.

ACKNOWLEDGEMENT
Authors acknowledge the immense help received from the scholars whose articles are cited and included in references of this manuscript. The authors are also grateful to authors / editors /publishers of all those articles, journals and books from where the literature for This article has been reviewed and discussed.

References:

1. Nikuradse, J. Laws of flow in rough pipes. NACA, Technical Memorandum 1292. November 1970.

2. Nunner, W. Heat transfer and pressure drop in rough tubes. VDI Forch 445-B 5-39 (1956); A.E.-R.E. Lib Trans. 786, 1958.

3. Dippery DF, Sabersky RH. Heat and momentum transfer in smooth and rough tubes at various Prandtl number. Int J Heat Mass Transfer 1963; 6:329–53.

4. Ravigururajan TS, Bergles AE.  General correlation for pressure drop and heat transfer for single phase turbulent flow in internally ribbed tubes. Augmentation of heat transfer in energy systems.HTD52. New York: ASME, 1985:9-20.

5. Han JC. Heat transfer and Friction in a channel with two opposite rib-roughened walls. Trans J Heat Transfer 1984; 106: 774– 81.

6. Han, J.C. L. R. Glicksman and W. M. Rohsenow, ?An investigation of heat transfer and friction for rib-roughened surfaces,? Int. J. of Heat and Mass Transfer. Vol. 21, pp.1143- 1156, 1978.

7. Lau SC, Kukreja RT, Mc Millin RD. Effects of V shaped rib arrays on turbulent heat transfer and friction of fully developed flow in a square channel. Int J Heat Mass Transfer 1991;34: 1605–16

8. Rajendra Karwa ,S.C. Solanki, J.S. Saini,Thermo-hydraulic performance of solar air heaters having integral chamfered rib roughness on absorber plates,Energy 26 (2001) 161–176.

9. Abdul-Malik Ebrahim Momin, J.S. Saini, S.C. Solanki, Heat transfer and friction in solar air heater duct with V-shaped rib roughness on absorber plate, International Journal of Heat and Mass Transfer 45 (2002) 3383–3396.

10. J.L. Bhagoria, J.S. Saini, S.C. Solanki, Heat transfer coefficient and friction factor correlations for rectangular solar air heater duct having transverse wedge shaped rib roughness on the absorber plate, Renewable Energy 25 (2002) 341–369.

11. Giovanni Tanda, Heat transfer in rectangular channels with transverse and V-shaped broken ribs, International Journal of Heat and Mass Transfer, Volume 47, Issue 2, 2004, Pages 229–243.

12. M.M. Sahu, J.L. Bhagoria, Augmentation of heat transfer coefficient by using 90° broken transverse ribs on absorber plate of solar air heater, Renewable Energy 30 (2005) 2057– 2073.

13. A.R. Jaurker, J.S. Saini, B.K. Gandhi, Heat transfer and friction characteristics of rectangular solar air heater duct using ribgrooved artificial roughness, Solar Energy 80 (2006) 895–907.

14. Apurba Layek , J.S. Saini, S.C. Solanki,Heat transfer and friction characteristics for artificially roughened ducts with compound turbulators, International Journal of Heat and Mass Transfer 50 (2007) 4845–4854.

15. Varun, R.P. Saini , S.K. Singal, Investigation of thermal performance of solar air heater having roughness elements as a combination of inclined and transverse ribs on the absorber plate, Renewable Energy 33 (2008) 1398– 1405.

16. S.K. Saini, R.P. Saini, Development of correlations for Nusselt number and friction factor for solar air heater with roughened duct having arc-shaped wire as artificial roughness, Solar Energy 82 (2008) 1118–1130.

17. R.P. Saini , Jitendra Verma, Heat transfer and friction factor correlations for a duct having dimple-shape artificial roughness for solar air heaters, Energy 33 (2008) 1277– 1287.

18. R. Kamali, A.R. Binesh, the importance of rib shapes effect on the local heat transfer and friction characteristics of square duct of ribbed internal surfaces, Heat and Mass Transfer 35 (2008) 1032–1040.

19. Varun , Amar Patnaik, R.P. Saini, S.K. Singal , Siddhartha, Performance prediction of solar air heater having roughened duct provided with transverse and inclined ribs as artificial roughness, Renewable Energy 34 (2009) 2914–2922.

20. Santosh B. Bopche , Madhukar S. Tandale, Experimental investigations on heat transfer and frictional characteristics of a turbulator roughened solar air heater duct, International Journal of Heat and Mass Transfer 52 (2009) 2834–2848.

21. K.R. Aharwal , Bhupendra K. Gandhi , J.S. Saini, Heat transfer and friction characteristics of solar air heater ducts having integral inclined discrete ribs on absorber plate, International Journal of Heat and Mass Transfer 52 (2009) 5970–5977.

22. Arvind Kumar , J.L. Bhagoria, R.M. Sarviya, Heat transfer and friction correlations for artificially roughened solar air heater duct with discrete W-shaped ribs, Energy Conversion and Management 50 (2009) 2106– 2117.

23. Apurba Layek, J.S. Saini, S.C. Solanki, Effect of chamfering on heat transfer and friction characteristics of solar air heater having absorber plate roughened with compound turbulators, Renewable Energy 34 (2009) 1292–1298.

24. Pongjet Promvonge, Heat transfer and pressure drop in a channel with multiple 60° V-baffles, International Communications in Heat and Mass Transfer 37 (2010) 835–840.

25. Rajendra Karwa, Kalpana Chauhan, Performance evaluation of solar air heaters having v-down discrete rib roughness on the absorber plate, Energy 35 (2010) 398–409.

26. V.S. Hans , R.P. Saini, J.S. Saini, Heat transfer and friction factor correlations for a solar air heater duct roughened artificially with multiple v-ribs, Solar Energy 84 (2010) 898– 911.

27. Giovanni Tanda, Effect of rib spacing on heat transfer and friction in a rectangular channel with 45° angled rib turbulators on one/two walls, International Journal of Heat and Mass Transfer 54 (2011) 1081–1090

28. Lanjewar ,J.L. Bhagoria, R.M. Sarviya, Experimental study of augmented heat transfer and friction in solar air heater with different orientations of W-Rib roughness, Experimental Thermal and Fluid Science 35 (2011) 986–995.

29. Brij Bhushan, Ranjit Singh, Nusselt number and friction factor correlations for solar air heater duct having artificially roughened absorber plate, Solar Energy 85 (2011) 1109– 1118.

30. Sukhmeet Singh, Subhash Chander, J.S. Saini, Heat transfer and friction factor correlations of solar air heater ducts artificially roughened with discrete V-down ribs, Energy 36 (2011) 5053-5064.

31. Atul Lanjewar, J.L. Bhagoria, R.M. Sarviya, Heat transfer and friction in solar air heater duct with W-shaped rib roughness on absorber plate, Energy 36 (2011) 4531-4541.

32. Brij Bhushan, Ranjit Singh, A review on methodology of artificial roughness used in duct of solar air heaters, Energy 35 (2010) 202-212.

35. R.J. Firth L. Meyer ,A comparison of the heat transfer and friction factor performance of four different types of artificially roughened surface, International Journal of Heat and Mass Transfer Volume 26, Issue 2, February 1983,175–18.

36. Xiufang Gao, Bengt Sunden, PIV measurement of the flow field in rectangular ducts with 60° parallel, crossed and Vshaped ribs Experimental Thermal and Fluid Science Volume 28, Issue 6, June 2004, Pages 639–653.

37. S.Y Son, K.D Kihm, J.C Han, PIV flow measurements for heat transfer characterization in two-pass square channels with smooth and 90° ribbed walls, International Journal of Heat and Mass Transfer, volume 45 issue24, 2002,pages 4809-4822

38. Zhang Y.M., Gu W Z, Han J C. heat transfer and friction in rectangular channels with ribbed or ribbed grooved walls,1994, transactions ASME journal of heat transfer, vol 116,number 1, pages58.

39. Han, J.C. and Park, J. S., ?Developing heat transfer in rectangular channels with rib turbulators,? Int. J. of Heat and Mass Transfer, Vol.3/1, pp.183-195, 1988.